Strange things happen at the one-two point.

One of my favourite proverbs, just because of the name. It sticks in your mind, and it should because it is so often vital for tight play in the corners. Here is the reference diagram. A one-two point is a point one line away from a corner, so there are eight of them on a board.
DIAGRAM 1
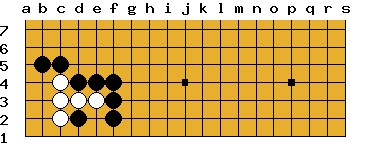
In this corner the one-two points are a2 and b1. It is so tempting for White to capture that Black stone and make one eye, but Black 2 (below) will kill the white group.
DIAGRAM 2
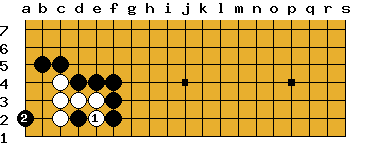
Black's move is called a 'monkey jump', and it is connected to the Black stone at b5. You might like to try to break that connection as White, just to prove it. Once you see that connection, you will realize that there is no space for White to make another eye. This monkey jump also takes the key one-two point.
DIAGRAM 3
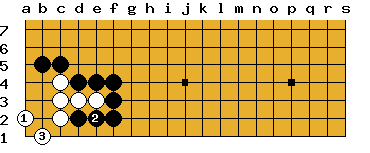
The only way for white to survive must start with White 1. This prevents the monkey jump and occupies the key one-two point. If Black saves his isolated stone at d2, taking the other one-two point secures the living group for White. White needs both one-two points in this example.
DIAGRAM 4
Here White tries to solidify the upper part, Black rescues the isolated stone, and White takes one of the one-two-points. But Black 4 steals the other one-two point and the White stones will die.
DIAGRAM 5
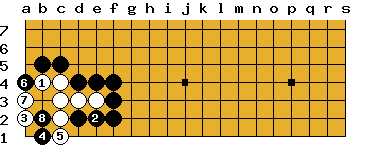
Please confirm that this sequence is the end for the White group.

Another example

DIAGRAM 6
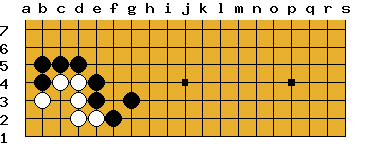
In Diagram 6, it looks as if a good attack for Black might be at b2, "squeezing" the white stone between two black stones. Let's see how that turns out.
DIAGRAM 7
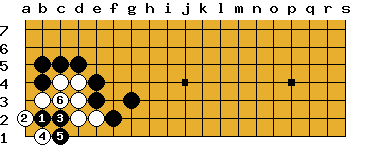
In fact after Diagram 7, Black can only get a ko fight started by capturing at a1. Black cannot kill unconditionally without winning the ko. Note that the White play at 2 is on the one-two point. Does Black have another way to attack? Of course, the one-two point!
DIAGRAM 8
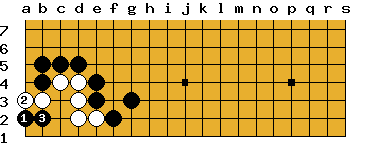
Here is how the attack starts. Now if White plays at c2, Black puts the two white stones in atari. Then a Black play at e1 ensures that White is dead.
DIAGRAM 9
The Go Proverbs Illustrated book goes on with four more examples, but I think you probably get the point. The one-two point is alwyas worth considering first.

Here is the final example, which I leave as a problem for you to solve. Black to play and kill unconditionally.